What does median mean?
A median is a statistical value that is equal to the middle value of an ordered set. In real estate, medians are used to estimate the general price of a home in a particular neighborhood or market. This is because the median is not affected by a few outlier home prices (very expensive or very cheap).
Other statistical methods used when calculating the typical price of a home is the average. To calculate the average you would add all numbers in a set and then divide the sum by the total number of all values in the set. The average can give better results only if the numbers in a set are close in value. That is the average is directly affected by the outliers, and for this reason, it does not give an accurate estimation in some cases.
Formula and Example of median
To calculate the median, you will need to order all the numbers in a set from the smallest to the biggest and then choose the middle value.
Lets assume that there are 5 houses sold in your neighborhood with the following prices: $100,000, $300,000, $125,000, $150,000, $700,000. To calculate the median, we will follow these steps.
Step1: Arrange prices from smallest to the highest
$100,000, $125,000, $150,000, $300,000, $700,000.
Step 2: Locate the middle number. This number will be your median.
$100,000, $125,000, $150,000, $300,000, $700,000. The middle number in our list is $150,000 since there are two numbers from either side of it.
This number tells you that a typical house in that location costs $150,000. You can also see that the median is not affected by expensive houses in the neighborhood ($300,000 and $700,000). This is why the median is more reliable compared to the average.
If we calculate the average, however, the results will be much different and unrealistic due to the outliers ($300,000, $700,000). To calculate the average, we will need to use the following formula.
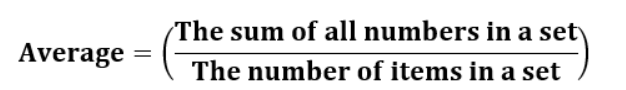
The first step is to calculate the sum of all values. Sum = $(100,000+125,000+150,000+300,000+700,000) =$137,5000.
Next, we will divide the sum by the total number in our set. Since there are 5 numbers in our set, we will divide the sum by 5. So, the average = $(1,375,000/5) = $275,000.
This value is unrealistic and does not reflect the typical price of a house in that area. This is because the majority of houses in that location cost far less than the average price.
How to calculate the median if you have an even number in your set?
There are times when the total number of values will be even. In this case, your calculations will be a little different from what we have seen in our example above.
To calculate the median, you will need to find the two middle number and then calculate their average.
What if we had 6 properties instead of 5? Let’s use our example and add one more price ($200,000) to make them even and then calculate the median. The new set will become: $100,000, $125,000, $150,000, $200,000, $300,000, $700,000.
If we attempt to calculate the middle number on this set, we will end up with the following:
$100,000, $125,000, $150,000, $200,000, $300,000, $700,000. There are two numbers ($150,000 and $200,000) in the middle and the median will be their average.
By using the average formula depicted above, the average will be ($150,000+$200,000)/2 = $175,000.
In other words, the typical price of a house in that location is $175,000.
The formula for average will be the same whether you have an even or odd numbers in a set.